Uncertainty in LIDT Specifications

This is Section 14.9 of the Laser Optics Resource Guide.

Laser-induced damage threshold (LIDT) specifications are not an absolute guarantee that damage will not occur below a certain value. Uncertainty in the LIDT value is introduced due to fluctuations in the test laser, the damage detection method, and under sampling the defects on the optic. This uncertainty leads to a confidence interval around the true damage probability as a function of fluence.

What is obtained from LIDT testing is a probability function based on a binomial distribution of experimental data. The confidence interval of damage occurring in a real setting can be determined using a Wilson score interval dependent on the probability function, and the number of observations made. The Wilson score interval (w) is a binomial proportion confidence interval given by:

(1)$$ w = \frac{1}{1 + \frac{z^2}{n}} \left( P + \frac{z^2}{2n} \pm z \sqrt{\frac{P \left( 1 - P \right)}{n}} + \frac{z^2}{4 n^2} \right) $$

n is the number of shots at each fluence level, P is the experimentally determined probability of damage, and z is the probit, or the quantile function of the standard normal distribution.¹ z corresponds to the desired confidence level. For example, z=1.96 for a 95% confidence level.

The ± sign in Equation 1 results in two possible values for the Wilson score interval. The higher value is the upper range of the confidence interval for whether or not damage will occur in a real application, while the lower value is the lower limit of the confidence interval of damage. Plotting w as a function of both n and P creates a 3D plot that represents a visual for determining the probability range of damage at a given confidence level (Figure 1). In Figure 1, at 10 shots per fluence level, it is only possible to know the probability of damage to approximately ±25%. If zero damage events are viewed over ten test sites, the worst-case probability of approximately 25% that damage would occur at the 11^th site. In order to know the probability of damage of better than ±5%, more than 100 shots are required at every fluence level. This high number of shots per fluence level is usually cost prohibitive, making simulation the ideal option for predicting the true behavior of an optic.

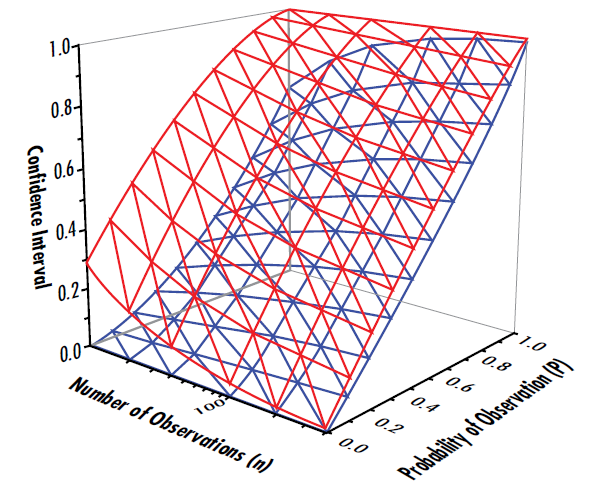
Figure 1: Confidence interval of the probability of damage of an optic, where the red surface is the upper limit of the confidence interval of whether damage will occur and the blue surface is the lower limit of the confidence interval

References

Wilson, Edwin B. “Probable Inference, the Law of Succession, and Statistical Inference.” Journal of the American Statistical Association, vol. 22, no. 158, 1927, pp. 209–212., doi:10.2307/2276774.

Previous Section Next Section